
Displaying 1477 - 1488 of 4948
Format results
-

-

-

Welcome and Opening Remarks
Lucien Hardy Perimeter Institute for Theoretical Physics
-

Gravitational Waves Experiments 3
Gabriele Vajente California Institute of Technology
PIRSA:18070039 -

-

-

Gravitational Waves Experiments 2
Gabriele Vajente California Institute of Technology
PIRSA:18070009 -

Gravitational Waves Experiments 1
Gabriele Vajente California Institute of Technology
PIRSA:18070038 -
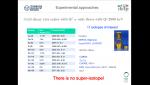
-

-

-
