
14533 - 14544 of 16890 Results
Format results
-

-
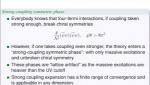
Lattice chirality: a mission (im)possible?
Yanwen Shang Citigroup Incorporated
-

Constraint free canonical GR using characteristic data
Michael Reisenberger Universidad de la Republica Uruguay
-

-

Quantum Non-Booleanity, Simultaneity and the Despatialization of Time
Kent Peacock University of Lethbridge
-

Quantum and Thermal decay in de Sitter space
Adam Brown Stanford University
-

Admissible transformations of quantum networks and their applications in quantum information processing
Giulio Chiribella University of Hong Kong (HKU)
-

-

Loop quantum gravity vertex for spin foam gravity
Roberto Pereira Aix-Marseille University
-

LQG spinfoam models: basic ideas and motivations
Jonathan Engle Aix-Marseille University
-

Asymptotic Expansion of the N=4 Dyon Degeneracy
Nabamita Banerjee Indian Institute of Science Education and Research, Bhopal
-

Tachyon Mediated Non-Gaussianity
Louis Leblond Pennsylvania State University