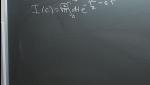
Mathematical Physics (PHYS 624) - Lecture 12
Carl Bender Washington University in St. Louis
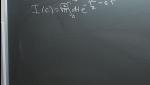
Carl Bender Washington University in St. Louis

Oliver Buerschaper Multiverse Computing (Germany)

Jaume Gomis Perimeter Institute for Theoretical Physics

Carl Bender Washington University in St. Louis

Philip Goyal State University of New York (SUNY)

Vincent Lam University of Queensland
Tim Ralph University of Queensland

Jaume Gomis Perimeter Institute for Theoretical Physics